In order to tie comments to specific locations in the book, I have used the available page ruler sheet to
identify lines in the text. A copy of that page ruler sheet can be printed from
The Index Sheet.
Chapter 1: Functions and Linear Models |
| 1.1 Functions fom the Numerical, Algebraic, and Graphical Viewpoints |
| Page | Line # | Notes |
| 37 | 19 |
Finding the distance between two points is actually important. I have the battleship games
both on the web, see the main battleship page, and on the calculators to
reinforce finding distances.
There is also a web page to explain
the battleship game in a bit more detail.
Finally, as of Jan. 23, 2014, there is a new
page that give a computational solution to the problem and an
implementation of that solution as a calculator
program.
|
| 41 | 3 |
The discussion of functions could be confusing. The book says forthe function n
that gives the number of facebook members for a value of t, years since 2004, that
t is the independent variable and n is the dependent
variable. But they just said that n is the function not a variable.
The book gets sloppy in moving from the function n
defined by the table on page 40, to n defined by reality, to n
by the graph of the table points, with the points connected by line segments. Note that there is no reason
to believe that the growth in each half-year
period follows those straing line segments.
|
| 42 | top |
The statement "we may be able to use an algebraic formula to calculate the function" is
absolutely wrong. The true statement would be
"we may have a mathematical (algebraic) formula that is a
function that closely approximates the real world function."
|
| 44 | 26 |
The use of the function
f(x) = -x2 + 20x +3
to approximate the number of iPod sales by years after 2004 and up to 2007
may be true, but there is no reason to expect this to be the case, nor is there
a reason to expect the model to be a quadratic function.
Look at the discussion about the proposed model for further information.
|
| 46 | 22 |
The book points out the function notation
f(x) = -x2 + 20x +3
and then the equation notation
y = -x2 + 20x +3
but the real picture if the noation
f = { (x,y) | y = -x2 + 20x +3 }
so that the function is defined as the set of
ordered pairs (x,y) such that y = -x2 + 20x +3, where
the vertiacl bar, | is read as "such that".
This is another example of how the exact science of mathematics is sometimes quite sloppy.
The book makes further note of this on page 58 at about line 23!
|
| 1.2 Functions and Models |
| Page | Line # | Notes |
| 57 | 38 |
The book mentions "slope" of the linear function, but the book does not define
"slope" until page 76.
|
| 58 | 36 |
Again, there is a sloppiness in the use of the character "m" in the equations here.
We should note:
|
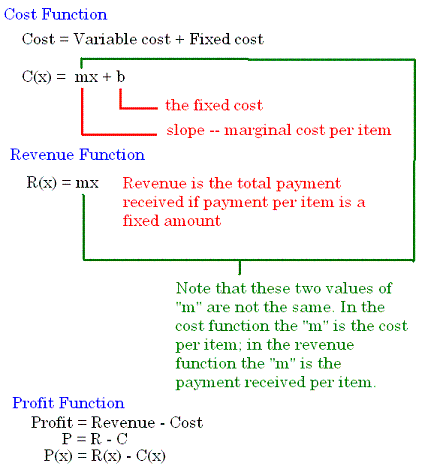
| 69 | top |
It does seem strange that the book does not offer a technology solution for 7b.
Therefore, look at Doing Example 7b for such a solution.
|
| 1.3. Linear Functions and Models |
| Page | Line # | Notes |
| 81 | 5 |
In part b the equation is given as 3x + 4y = 5. This is the Standard Form
for a linear equation (as opposed to the slope-intercept form: y=mx+b). The general statement of the standard form is
Ax + By = C where A, B, and
C are integers and A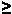 0.
Note that the B in the standard form and the b in the slope-intercept form are not
the same thing. If the B in the standard for is not 0, then we can manipulate
the Standard form into the slope-intercept form as:
Ax + By = C
By = -Ax + C
y = (-A/B)x + (C/B)
which gives m as (-A/B) and b as (C/B).
|
| 81 | all |
The book has four parts to Example 2. These cover many, but not all of the forms of questions usually posed about finding
linear equations. Here is a more complete list
- You are given the slope and the y-intercept, find the equation of the line;
- You are given the slope and a point on the line, but not the intercept, find the equation of the line;
- You are given two points on the line, find the equation of the line;
- You are told that the line is horizontal and given a point on the line, find the equation of the line;
- You are told that the line is vertical and given a point on the line, find the equation of the line;
- You are told that the line is parallel to a line given in slope-intercept form, and that the
new line contains a point, find the equation of the line;
- You are told that the line is perpendicular to a line given in slope-intercept form and that
the line contains a point, find the equation of the line;
- You are told that the line is parallel to a line given in standard form and that the new line
contains a point, find the equation of the line;
- You are told that the line is perpendicular to a line given in standard form and that the new line
contains a point, find the equation of the line.
Many of these become quite mechanical solutions, some to the point of just writing out the answer, others lending themselves to
a straight forward program of steps.
The web page Solving for Linear Equations goes over all
of this, including presenting two programs
for the TI-83 family, slope and slope1, that implement the programed steps.
|
| 1.4 Linear Regression |
| Page | Line # | Notes |
| 95 | 10 |
The explanation in the book hits most of the
points that I consider important. I do have a separate
web page giving another regression example from a computational perspective.
Then the web page Linear Regression on a TI-83 walks you
through another example, this time using the TI-83 to do all the work.
|