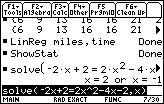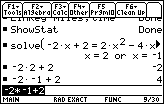Please note that the calculator used to produce the graphs on this page had just been used
to produce the graphs and screen images from the web page 238901.htm.
As a result there is some residual material on this calculator.
Figure 1
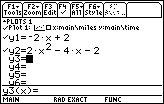
| Figure 1 is taken from the y= screen on a TI-89 after we have defined
the two functions. The first function is a straight line, and the second is a quadratic which will
be graphed as a parabola. |
Figure 2
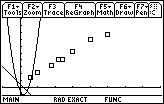
| Pressing   moves the display to Figure 2. Here we see some unexpected items.
For one thing, the graph shows a number of points that are not related to this problem at all.
Second, the WINDOW settings for the graph are not obvious.
moves the display to Figure 2. Here we see some unexpected items.
For one thing, the graph shows a number of points that are not related to this problem at all.
Second, the WINDOW settings for the graph are not obvious.
|
Figure 3
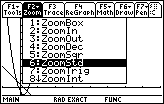
| We will use the ZOOM feature to change the WINDOW settings to the standard values.
To do this we press  to open the window shown in Figure 3.
Then, to select ZoomStd we press to open the window shown in Figure 3.
Then, to select ZoomStd we press  . This will produce Figure 4. . This will produce Figure 4. |
Figure 4
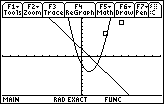
| In Figure 4 we have the more usual Window settings, namely both x and
y range between – 10 and 10.
Two of the plotted points remain visible on the new WINDOW.
They are left over from earlier work on this calculator. |
Figure 5

| To generate Figure 5 we need to return to the y= screen.
We can press   to do this. On this screen we note that Plot 1 is
not only defined, but it is active, as noted by the check mark
to the left of Plot 1. Furthermore, we have used the
to do this. On this screen we note that Plot 1 is
not only defined, but it is active, as noted by the check mark
to the left of Plot 1. Furthermore, we have used the
 key to move the highlight to Plot 1. key to move the highlight to Plot 1.
|
Figure 6

| We will unselect Plot 1 by
pressing the  key. Note that the check mark has been removed.
The plot remains defined, but it will not be graphed. key. Note that the check mark has been removed.
The plot remains defined, but it will not be graphed. |
Figure 7
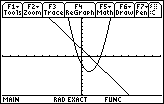
|   returns the calculator to the graph
screen shown in Figure 7. returns the calculator to the graph
screen shown in Figure 7.
Our purpose here is to use the Intersection
command to find the two points of intersection.
We could modify the WINDOW settings to have the graph come close
to the one presented in the text, but that will not
be necessary.
Then, use  to select the Math
tab at the top of the window. to select the Math
tab at the top of the window. |
Figure 8
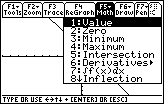
| From the options shown in Figure 8, we want to choose item
5: Intersection. Press  to make that choice. to make that choice.
|
Figure 9
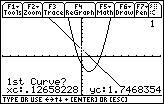
| Iintersection goes through a number of steps. First, we need to choose the two curves to
use. It is possible that we would have more than 2 curves graphed at the same time.
The calculator wants us to choose the two graphs to use. This seems a bit superfluous
here given that there are only two functions on this graph. However, we will
have to play along with the calcualtor.
The TI-89 is proposing the first function as one of the two curves.
We press  to accept that choice. to accept that choice.
|
Figure 10
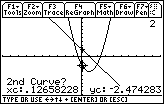
| In Figure 10 the TI-89 proposes the second function as the the second curve.
Again, press  to accept that choice. to accept that choice. |
Figure 11
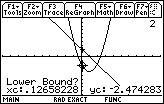
| The TI-89 will look for a solution, a point of intersection,
within a specified domain. In Figure 11, the TI-89 is proposing a left or Lower
Bound for that specified domain. Assuming that we want to find the left point of
intersection, the proposed point will not do.
Therefore, we use the  key to move the left or Lower Bound to the left of the point of
intersection that we can see on the screen. That change is reflected in Figure 12.
key to move the left or Lower Bound to the left of the point of
intersection that we can see on the screen. That change is reflected in Figure 12. |
Figure 12
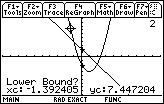
| The pointer on the screen is to the left of the
desired point of intersection.
We press  to accept that lower bound. to accept that lower bound. |
Figure 13
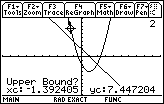
| The next task is to specify a bound to the right of the point of intersection.
In Figure 13 the calculator is proposing a point that is on the wrong side of the intersection.
We use the  key to move the pointer to the right side
of the intersection. key to move the pointer to the right side
of the intersection. |
Figure 14
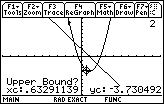
| Now the pointer is to the right of the intersection.
This gives an Upper Bound to the domain of values. We press
 to accept this point. to accept this point.
Before leaving Figure 14, please note that the TI-89 has left a small marker to indicate the
location of the Lower Bound. That marker is obscured
by the graph of the parabola. A better example will appear in Figure 18.
|
Figure 15
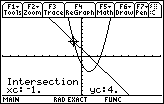
| The TI-89 has determined that the left point of
intersection is (– 1,4).
Now we want to obtain the coordinates of the other point of intersection.
To do this we step through the same sequence of screens, but changing our Lower Bound
and Upper Bound
so that they bracket the right point of intersection.
|
Figure 16
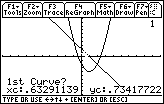
| We press  to open the Math
menu. We press to open the Math
menu. We press  to select 5: Intersection.
The calculator proposes the first curve.
We press to select 5: Intersection.
The calculator proposes the first curve.
We press  to accept that choice. to accept that choice.
|
Figure 17
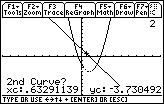
| The calculator proposes the second curve. Again,
 accepts that choice. accepts that choice. |
Figure 18
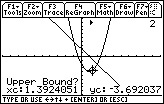
| The TI-89 proposed a Lower Bound. We pressed
 to accept that Lower Bound.
That action left a marker on the screen to indicate the location of the Lower Bound.
The TI-89 then proposed an Upper Bound. That proposed point is shown in Figure 18. to accept that Lower Bound.
That action left a marker on the screen to indicate the location of the Lower Bound.
The TI-89 then proposed an Upper Bound. That proposed point is shown in Figure 18.
|
Figure 19
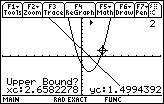
| We use the
 key to position the proposed Upper Bound to the right of the other
intersection.
Then press key to position the proposed Upper Bound to the right of the other
intersection.
Then press  to accept that Upper Bound. to accept that Upper Bound.
|
Figure 20
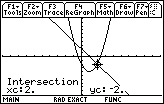
| Intersection has done its work. The right point of intersection
is identified as (2,– 2).
|
Figure 21
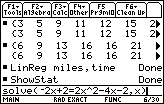
| The TI-89 is a powerful tool. The Figures given above demonstrated the use of the
Intersection command for finding points of intersection on from two graphed functions.
Those functions were given as
f(x) = – 2x + 2
g(x) = 2x2 – 4x –2
We could just as easily have asked the calculator to solve
– 2x + 2 =
2x2 – 4x –2
Figure 21 shows the command used to do exactly that.
|
Figure 22
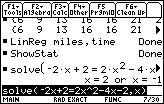
| Figure 22 gives the two values of x that
solve the previous equation.
|
Figure 23
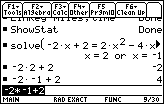
| We can use the definitions of the two functions
to find the corresponding y values. |
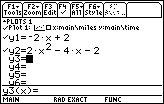


 moves the display to Figure 2. Here we see some unexpected items.
For one thing, the graph shows a number of points that are not related to this problem at all.
Second, the WINDOW settings for the graph are not obvious.
moves the display to Figure 2. Here we see some unexpected items.
For one thing, the graph shows a number of points that are not related to this problem at all.
Second, the WINDOW settings for the graph are not obvious.

 to open the window shown in Figure 3.
Then, to select ZoomStd we press
to open the window shown in Figure 3.
Then, to select ZoomStd we press  . This will produce Figure 4.
. This will produce Figure 4. 


 to do this. On this screen we note that Plot 1 is
not only defined, but it is active, as noted by the check mark
to the left of Plot 1. Furthermore, we have used the
to do this. On this screen we note that Plot 1 is
not only defined, but it is active, as noted by the check mark
to the left of Plot 1. Furthermore, we have used the
 key to move the highlight to Plot 1.
key to move the highlight to Plot 1.

 key. Note that the check mark has been removed.
The plot remains defined, but it will not be graphed.
key. Note that the check mark has been removed.
The plot remains defined, but it will not be graphed.

 returns the calculator to the graph
screen shown in Figure 7.
returns the calculator to the graph
screen shown in Figure 7.
 to select the Math
tab at the top of the window.
to select the Math
tab at the top of the window.
 to make that choice.
to make that choice.

 to accept that choice.
to accept that choice.

 to accept that choice.
to accept that choice.
 key to move the left or Lower Bound to the left of the point of
intersection that we can see on the screen. That change is reflected in Figure 12.
key to move the left or Lower Bound to the left of the point of
intersection that we can see on the screen. That change is reflected in Figure 12. 
 to accept that lower bound.
to accept that lower bound. 
 key to move the pointer to the right side
of the intersection.
key to move the pointer to the right side
of the intersection. 
 to accept this point.
to accept this point.


 to open the Math
menu. We press
to open the Math
menu. We press  to select 5: Intersection.
The calculator proposes the first curve.
We press
to select 5: Intersection.
The calculator proposes the first curve.
We press  to accept that choice.
to accept that choice.

 accepts that choice.
accepts that choice. 
 to accept that Lower Bound.
That action left a marker on the screen to indicate the location of the Lower Bound.
The TI-89 then proposed an Upper Bound. That proposed point is shown in Figure 18.
to accept that Lower Bound.
That action left a marker on the screen to indicate the location of the Lower Bound.
The TI-89 then proposed an Upper Bound. That proposed point is shown in Figure 18.

 key to position the proposed Upper Bound to the right of the other
intersection.
Then press
key to position the proposed Upper Bound to the right of the other
intersection.
Then press  to accept that Upper Bound.
to accept that Upper Bound.