TI-83: Complex 3 equation 2 variable situation, where there is a solution

The main page for solving systems of linear equations on the TI-83 and TI-83 Plus.
The previous example page covers a Complex 3 equations in 2 variables, 2 parallel, no unique solution.
The next example page covers a Complex 3 equation 2 variable situation, where two lines are identical and there is a solution.

WARNING: The TI-83 and TI-83 Plus are almost identical in terms of the material presented here. The major difference is the labels that are on certain keys. The TI-83 has a

key, whereas on the TI-83 Plus requires 2 keys to achieve the same result, namely, the

key. The text below will be done from the perspective of the TI-83. That is, all reference to the MATRIX key will be demonstrated via the

key. If the user has a TI-83 Plus then the key strokes should be

. To save some space, and to ignore this difference, the numeric keys (the gray ones) have been changed in some places to show the key face, as in

. In addition, the

key may be shown as

and the

key may be shown as

, again to save space.

The problem we will use on this page is 3x + 4y = 43
5x - 2y = 11
11x - 13y = -36 As was the case in the previous example, we have just two variables, but we have 3 equations. Our use of the rref function has been in situations where the number of equations is equal to the number of variables. In a real sense, we have an extra equation here. If we only had two equations then we could use rref to find their point of intersection. Let us move ahead in that direction. At first we will restrict our analysis to the first two equations: 3x + 4y = 43
5x - 2y = 11

Figure 1	By now we are aquainted with the steps of getting through the MATRIX menu to EDIT [A], change the dimensions if need be, and then enter the values of the coefficients and constants from our equations. This has been done in Figure 1.
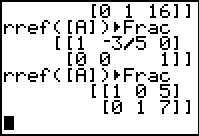
Figure 2	In Figure 2 we have quit the matrix editor, recalled the command that we want to use, and performed the command. The resulting matrix yields the solution x=5 and y=7.
Figure 3	Having used the first and second equation and having found a solution, we do have the option of just seeing if the solution works in the third equation. We could go through the following restatement,substitution, and simplification: 11x - 13y = -36 115 - 137 ?= -36 55 - 91 ?= -36 -36 = -36 where the "?=" is there because as we do the steps we do not know if it will work. This shows that the point (5,7) does indeed satisfy the third equation and, therefore, that point is a solution to all three equations. At the same time, we could, as is shown in Figure 3, modify the matrix to hold the third equation and one of the other two, in this case the first, and then move to Figure 4 to recall and perform the rref function to transform those two equations into a solution.
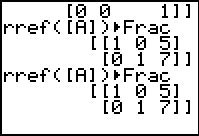
Figure 4	Indeed, as we expected the solution point for the first and third equations is the same (5,7).
Figure 5	In Figure 5 we return and, just for completeness, modify the matrix to hold the second and third equations.
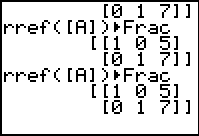
Figure 6	Performing the rref function yet again we get, as expected, the same result. Indeed, all three equations contain the point (5,7).
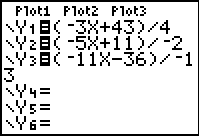
Figure 7	In order to take a look at the three equations we move to the Y= screen. All three equations have been put into the appropriate form and entered into that screen.
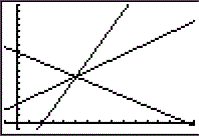
Figure 8	Move to these functions. The result is shown in Figure 8 which is using the WINDOW settings from a previous example. This graph illlustrates the situation where three lines intersect in a single point.