| Symbol | Meaning | Alternate Keyboard Symbols |
| < | less than | < |
| ≤ | less than or equal to | < = |
| > | greater than | > |
| ≥ | greater than or equal to | > = |
| ≠ | not equal | != or < > |

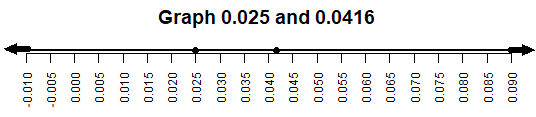
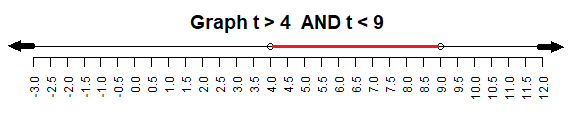
 .
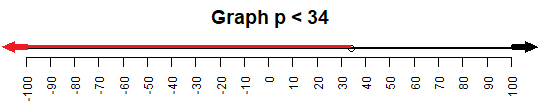
.
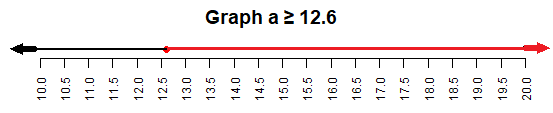
 , indicates that 12.6 is a possible value for
a ≥ 12.6.
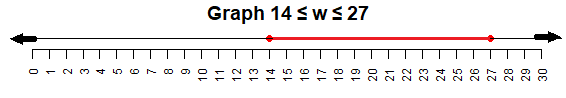
, indicates that 12.6 is a possible value for
a ≥ 12.6.
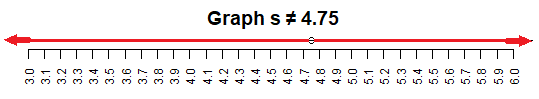
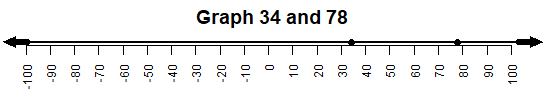
 , indicates that 4.75 is not a possible value for
s ≠ 4.75.
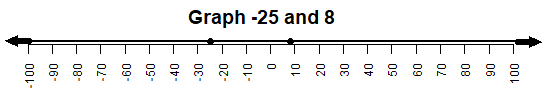
, indicates that 4.75 is not a possible value for
s ≠ 4.75.