Figure 1
|
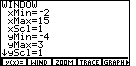
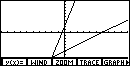
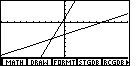
Figure 1 shows the result of pressing the  key
on my TI-86 calculator. The actual output depends on the previous state of the
calculator. In this example, it would appear that no functions have been defined and
that the window (i.e., range) settings are not at all standard.
|
Figure 2
| Press the  key
to see the "y(x)=" list. On this calculator that list is empty. The
calculator is ready to receive the first function definition. |
Figure 3
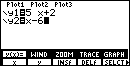
| To produce Figure 3 we have pressed the
 and  keys
to create the first function. Then we press the
 key to move to the second function.
That function is created by the
  and
 keys.
|
Figure 4
| Having entered the functions in Figure 3, we press
 
to select the GRAPH command from the top menu. The result is shown in Figure 4.
We can see the graphs of the two straight lines in Figure 4, but this graph does not appear
as does the graph in the text. We will look at the WINDOW settings to see
the current values and to change those values if need be.
|
Figure 5
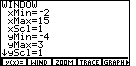
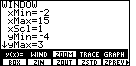
| To open the WINDOW menu we press the
 key to select the WIND command (RANGE on a
TI-85). The result is shown in Figure 5. Clearly these are not the
values that we want
to be using. Rather than type all new values, let us use the ZOOM menu
to change the WINDOW settings. To do this,
press the  key to select the ZOOM option.
This will open a submenu, as is shown in Figure 6. |
Figure 6
| Figure 6 shows the start of the ZOOM submenu. We will opt for the
ZSTD option by pressing  .
This will set the xMin value to -10, xMax to 10, xScl to 1,
yMin to -10, yMax to 10, and yScl to 1. It also moves us back to
graph mode and a new graph, see Figure 7, will be drawn. |
Figure 7
| In Figure 7 we have the new graph that uses the new WINDOW settings.
It still does not seem to correspond to the figure in the book. First, the x-axis
in Figure 7 needs to be raised. We can do this by making the yMin value more negative.
Second, the "tick" marks on the y-axis are too close together. We can alter
this by increasing the value assigned to yScl. |
Figure 8
|
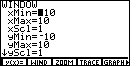
We move to Figure 8 by presing the key to open the WINDOW
settings. A review of Figure 8 shows that we have indeed established the ZSTD settings
described above.
|
Figure 9
| Figure 9 shows the WINDOW settings screen after we have used the cursor keys
to move down to the yMin line, and then we entered a new value for yMin, namely, -20.
Then we moved to the yScl line and changed that value to be 2. |
Figure 10
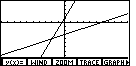
| Now, to get to Figure 10 we press the
key to do another graph.
This graph looks similar to the graph in the textbook. However, the graph in
the text tells us the x and y coordinate of the point of intersection of the two lines.
We need to find the command to get the calcualtor to find that point.
A review of the menu at the bottom of Figure 10 does not give any hint of
our desired command. However, the small arrow at the right end of the menu indicates that
there are more items. We press the
 key to see additional items in the menu.
|
Figure 11
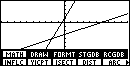
| Figure 11 shows the next five items in the menu. Now we have a interesting
choice, namely, the MATH submenu. Press
to select that choice. |
Figure 12
| The MATH submenu is displayed in Figure 12. Again, it does not seem to have the
command that we desire. We press the
key to see additional items in the MATH submenu.
These can be seen in Figure 13.
|
Figure 13
| The middle option in the MATH submenu is ISECT, a plausible
abbreviation for INTERSECTION. We press the
key to select the ISECT option. |
Figure 14
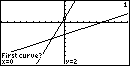
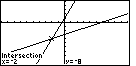
| As a result of selecting the ISECT option, Figure 14 shows us that the
calculator is proposing that our line for y(x)=5x+2 be our first curve. We can
examine the graph in Figure 14 and we will see that at the point, x=0, y=2, the calcualtor
has displayed a special symbol. That is how the calculator identifies the particular
line that it is proposing to use. We can press the
key to accept that proposal.
This will move the graph to Figure 15. |
Figure 15
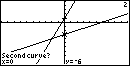
| Now we need to select the second curve. The calculator is proposing the other line, y(x)=x-6,
for the second curve. The calculator is making this proposal by displaying its
flashing sign on a point, x=0, y=-6, on that line.
Again, we will accept the proposal by pressing the
key. |
Figure 16
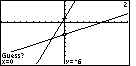
| In Figure 16 the calculator has been given the two curves, and now it wants a guess,
a starting point. In fact, the calcualtor offers the same point (0,-6) as that starting
point. We can accept that point as our guess by pressing
the key. |
Figure 17
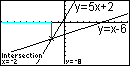
| As a result of all of our efforts, the calculator display shifts to
Figure 17. In that Figure, the calculator has identified the point of intersection
of the two lines, namely at the point (-2,-8). This graph is remarkably similar to the
graph that is given in the textbook. The only difference is that the textbook version contains the
equations of the two lines. A closer examination of the graph in the textbook shows that these
equations were pasted into the book; after all, the equations in the book appear in
a completely different font. We too can doctor a picture. We have done that in Figure 18. |
Figure 18
| You can not produce Figure 18 directly from the calculator. The equations have been
added to this image. In addition, we have added a red line from the point of
intersection straight up to the x-axis.
In additon, we have replaced the x-axis from directly above the point of intersection all the
way to the left by a light blue line. This represents the
x-values where the line y(x)=5x+2 is below the line y(x)=x-6. In effect, this is the
set of points where 5x+2 < x-6 which was the original problem.
|